Dimensional Analysis

Introduction

Conversion Factors and Their Reciprocals

Use of Conversion Factors: Some Examples

Developing the Units Path

Summary and Helpful Hints

 

Introduction

Example 1: Suppose you want to convert $100 in U.S. dollars to Canadian dollars. You know that the exchange rate is currently $1.50 Cdn. for every $1.00 U.S. How much Canadian cash will you get?

Solution. You probably solved this in your head: you'll expect to receive $150 Canadian for your $100 U.S. But think carefully! This isn't intuitively obvious, you had to learn how to do it. The steps you probably followed, so fast you didn't realize, were these:

1. You recognized that you were starting with $100 U.S. (the starting quantity)
2. You got a conversion factor from the English sentence that tells you the exchange rate: the conversion factor is

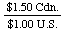
3. You then multiplied the starting quantity by the conversion factor to get your answer (the desired quantity):

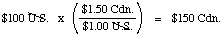
This gives us a method for solving many problems that aren't so obvious as converting U.S. to Canadian dollars.

The Dimensional Analysis Method Identify the starting quantity. Write it down. (In the above example, it was $100 U.S.) Decide what units (dimensions) you need for your answer. Develop a path that gets you from your starting units to the units you need. Write down the conversion factor between the units. Following the "units path," multiply your starting quantity by one or more conversion factors so that you finally obtain the units you need. All the unwanted units will cancel each other when you multiply. To put it in simpler terms, we want to know how to get from what we are GIVEN (the starting quantity) to what we WANT TO KNOW (the desired quantity)

Dimensional analysis is sometimes called the factor-label method or the factor-unit method. That is because it involves two quantities: a mathematical proportionality that is thefactor and an expression of the units, or label.

Conversion Factors and Their Reciprocals

Every conversion factor has a reciprocal that can be used for the opposite conversion. To convert $U.S. to Canadian dollars, we used the factor

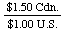
However, if we wanted to convert Canadian dollars to U.S. dollars, we would use the reciprocal of the above factor, or

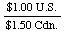
So, if we had $500 Cdn., we could determine how much U.S. money we could get in exchange:

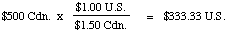
Below are some other conversion factors you will have seen in your studies of chemistry.

Conversion Factor	Reciprocal of Conversion Factor
10 mm / cm	1 cm / 10 mm
453.6 g / lb	161 lb / 453.6 g
6.022 x 1023 atoms Cu / mol Cu	1 mol Cu / 6.022 x 1023 atoms Cu
63.546 g Cu / mol Cu	1 mol Cu / 63.546 g Cu
2 mol NaOH / mol H2SO4	1 mol H2SO4 / 2 mol NaOH
36.9 g Fe2O3 / 100 g ore	100 g ore / 36.9 g Fe2O3

Use of Conversion Factors: Some Examples

Example 1. (a) Write the reciprocal for the following conversion factor: 1 mol NaOH / 40.0 g NaOH. (b) Which of these (the original factor or its reciprocal) do we use if we want to change the mass of NaOH (49.7 g) to moles?

Answer: (a) 40.0 g NaOH / mol NaOH
(b) the original factor: (49.7 g NaOH) x (1 mol NaOH / 40.0 g NaOH) gives units of moles NaOH

Example 2. (a) Write the reciprocal for the following conversion factor: 3 atoms S / molecule P₄S₃. (b) Which of these (the original factor or its reciprocal) do we use if we want to calculate how many molecules of P₄S₃ can be made from a 1200 S atoms?

Answer: (a) 1 molecule P₄S₃ / 3 atoms S
(b) the reciprocal factor: (1200 S atoms) x (1 molecule P₄S₃ / 3 atoms S) gives units of molecules of P₄S₃

Example 3. (a) Write the reciprocal of the following conversion factor: 3 mol AgCl / mol CrCl₃. (b) Which of these (the original factor or its reciprocal) do we use if we want to calculate how many moles of AgCl can be prepared from 0.1250 moles of CrCl₃?

Answer: (a) 1 mol CrCl₃ / 3 mol AgCl
(b) the original factor: (0.1250 mol CrCl₃) x ( 3 mol AgCl / mol CrCl₃) has units of moles AgCl

 

Example 4. (a) Write the conversion factor for "36.3% chocolate chips in Chips Ahoy cookies," then write its reciprocal. Which of these (the original or its reciprocal) do we use to calculate how many cookies have to be processed to yield a 500. g of chocolate chips?

Answer: (a) conversion factor is 36.3 g chocolate chips / 100 g cookies; reciprocal is 100 g cookies / 36.3 g chocolate chips

(b) the reciprocal factor: (500. g chocolate chips) x (100 g cookies / 36.3 g chocolate chips) has units of mass of cookies.

 

Developing the Units Path: Extended Examples

Example 1. You have 45.5 grams of iron, Fe. What mass (in g) of Fe₂O₃ can you make if you react the iron with oxygen? The balanced equation is

4 Fe + 3 O₂ ---> 2 Fe₂O₃

Analysis: We want to know how we can get from what we are given (45.5 g Fe) to what we WANT (mass of Fe₂O₃). Now you know about chemistry and balanced equations, so you know that the MOLE is the important unit here, and we'll have to work through moles. If we want to get from mass of Fe to mass of Fe₂O₃, we'll have to have a bunch of conversion factors since no single factor that we've been given can get us there.

The balanced equation gives us this conversion factor that relates the product to the reactant: 4 mol Fe / 2 mol Fe₂O₃. We have two other conversion factors, the molar masses, that allow us to go from masses to moles and vice versa. We also have the reciprocals of these factors.

The sequence of operations is this:

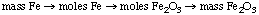
THIS SEQUENCE IS IMPORTANT! It may take a while to figure out, and you may have to play with conversion factors to realize just how you'll get from one step to another. But by now you'll know that mass/mole conversions are made through the conversion factor molar mass or its reciprocal. And the balanced equation gives you the connection between the moles of reactants and products.

So: we begin with 45.5 g Fe, and use the above sequence with its conversion factors chosen so that all the units cancel except "g Fe₂O₃" which is what we wanted to find:

 

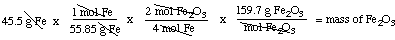
Example 2. You are a chemist in an ore-processing plant that produces iron metal. You are told that the new shipment of ore coming in contains 56.3% Fe₂O₃. Your company asks you: how many tonnes of iron can be obtained from 5.00 x 10² tonnes of ore? (1 tonne = 1000 kg).

Analysis. You are given the amount of ore that has to be processed. That will be your starting point for this question. Once again, you'll need to work through moles. You'll also have to turn "56.3% Fe₂O₃" into a conversion factor:

56.3 % Fe₂O₃ = 56.3 g Fe₂O₃ / 100 g ore

The unit path is then

mass of ore ---> mass of Fe₂O₃ ---> moles Fe₂O₃ ---> moles Fe ---> mass Fe

So we begin with the mass of ore (5.00 x 10² tonnes) and proceed in serval steps:

Step 1: mass of ore to mass of Fe₂O₃ in grams
Step 2: mass of Fe₂O₃ to mass of Fe
Step 3: mass of Fe in grams to mass in tonnes

Step 1:

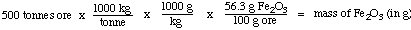
Step 2:

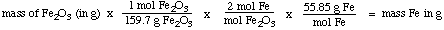
Step 3:

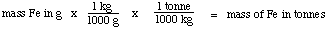
Summary

Learning this method takes practice, but it helps if you remember the basic process:

• Write down what you are GIVEN and its units
• Write down what you are ASKED TO FIND and its units
• Determine the conversion factors that might be needed to develop the units path beteween what you are given and what you are asked to find.
• Finally, multiply what you are given by the conversion factors (or their reciprocals if necessary to make the units cancel). If the units of your final answer are correct, and your conversion factors are correct, the answer you obtain almost certainly has to be the correct answer.

HELPFUL HINTS:

• In the Dimensional Analysis Tutorial that you did in the first lab, the conversion factors were referred to as "fractions" (because they are fractions: one number with its units over another number with its units).
• In Section 1 of the Tutorial, "Translation of Statements into Fractions," you are asked to write the fraction in two ways. These two ways correspond to the conversion factor and its reciprocal.

This page is http://chemiris.labs.brocku.ca/~chemweb/courses/chem180/Dimensional_Analysis.html
Created October 10, 2000 by M. F. Richardson
© Brock University, 2000