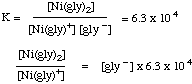
= 0.10 x 6.3 x 104 = 6.3 x 103
1. Logarithms for the stepwise formation constants of nickel(II) with the glycinate ion (NH2CH2CO2-) are as follows: log K1 = 5.77, log K2 = 4.80, log K3 = 3.61.
(a) Write an equation for the reaction for which K2 is the equilibrium constant. Answer: K2 refers to the reaction to which the second glycinate is added to a complex already containing one glycinate.
Ni(gly)+ + gly - ---> Ni(gly)2
(b) If the concentration of the glycinate ion is 0.10 M, what is the ratio of [Ni(gly)2] to [Ni(gly)+]? Answer: write the equilibrium constant for the reaction in part (a), and substitute for the glycinate concentration given.
(c) Write an equation for the reaction for which Beta2 is the equilibrium constant. Answer: the overall formation constant Beta2 refers to the reaction in which the metal ion reacts with 2 ligands: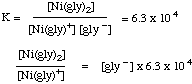= 0.10 x 6.3 x 104 = 6.3 x 103
Ni2+ + 2 gly - ---> Ni(gly)2(d) Use the values of the stepwise constants and calculate the value of Beta3. Answer: the overall formation constant Betan can be shown to be equal to the product of the stepwise constants up to Kn: Betan = K1 x K2 x K3 x .... x Kn. So,
Beta3 = K1 x K2 x K32. The logarithm of the overall equilibrium constant for the reaction of [Zn(H2O)6]2+ with 2 moles of ethylenediamine is 10.8 . The logarithm of the overall equilibrium constant for the reaction of [Zn(H2O)6]2+ with 1 mole of 2,2',2"-triaminotriethylamine, N(CH2CH2NH2)3, is 14.6.= 1.5 x 1014
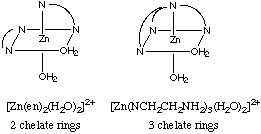
(a) Sketch the structure of [Zn(en)2]2+. How many
chelate rings does it have?
(b) Sketch the structure of [ZnN(CH2CH2NH2)3]2+.
How may chelate rings does it have? Answer:
(c) Determine the equilibrium constant for the following reaction:
[Zn(en)2]2+ + N(CH2CH2NH2)3 ---> [ZnN(CH2CH2NH2)3]2+ + 2 enAnswer: Write the equilibrium constant for the above reaction. Then think about how you can get it in terms of the data you are given. Since you have formation constants given for each complex, and formation constants have to contain the concentration of the metal ion, try multiplying through by [Zn2+]/[Zn2+]. Then group terms as shown below. (It might help to write the chemical equations, and then the equilibrium constant expressions, for the formation constants given in this question.)
(d) Is this reaction thermodynamically favorable? How could you have predicted this? Answer: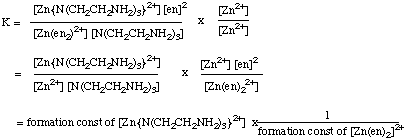
= (4.0 x 1014) x (1/6.3 x 1010) = 6.3 x 103
Yes, it is thermodynamically favorable since the equilibrium constant is greater than one. It could have been predicted since the N(CH2CH2NH2)3 complex has more chelate rings than the complex with two ethylenediamine molecules (both complexes have the same number of water molecules replaced by the ligands). Also, the reaction in part (c) has two particles reacting to produce three particles, and this should be entropy-favored.
3. The logarithm of the overall equilibrium constant for the reaction of [Ni(H2O)6]2+ with one mole of beta-alanine anion, NH2CH2CH2CO2-, is 4.63. The logarithm of the overall equilibrium constant of [Ni(H2O)6]2+ with one mole of the glycinate ion is 5.77.
(a) Make a sketch to show the chelate rings formed by the two ligands. See Question 1 for the structure of the glycinate anion. Answer
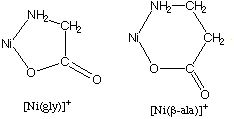
(b) Which is more stable chelate ring system: a 5-membered chelate ring or a 6-membered chelate ring? Answer
5-membered chelate ring is more stable here, since the formation constant of the glycinate complex is greater than the formation constant of the beta-alanine complex.4. Hints. See any first-year textbook for sketches.
The first hydration shell for a cation is formed when the negative end of the water dipole is attracted to the metal ion. Hexaaquo metal ions are frequently formed, but small ions such as Be2+ may have only four water molecules in the first hydration shell, and large ones such as La3+ may have 8 or more.The first hydration shell for the anion is formed when the positive end of the water dipole is attracted to the anion; H-bonding usually occurs to the anion.
If charges are high enough on cation and anion, additional hydration shells can be formed, but this would be hard to draw with any accuracy.
5. The pKa for the first hydrolysis of Y(III) is 7.7. (a) Write the equation for the chemical reaction for which this is the equilibrium constant. (b) Calculate the H3O+ concentration in a solution that contains 0.20 M Y(III). Hint: this question is exactly like finding the H3O+ concentration in a solution of any weak acid such as acetic acid, CH3CO2H.
Y(H2O)83+ + H2O ---> Y(H2O)7(OH)2+ + H3O+
0.20 -x x x
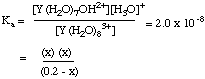
This quadratic equation can be solved by the usual methods.
Questions 6, 7, 8. See section 2.3 in Wulfsberg.
This page is http://chemiris.labs.brocku.ca/~chemweb/courses/chem232/CHEM_2P32_Assign_5.html
Last revised: February 22 2001 by M. F. Richardson
© Brock University, 2001